Semendyaev S.
Abstract: In this paper a solid system with rotating eccentrics as internal movers is investigated. The system moves with dry friction. Massive eccentric converts its rotational motion into rotation of the body(tripod). The spinning of the tripod with the pendulum as internal mover is considered in mathematical model and experiment; the resulting system is presented in a convenient form for further investigation by numerical methods.
I. INTRODUCTION
There is growing interest in mechanical systems moving due to internal movement of masses without using external movers, such as wheels, legs or tracks. A new class of mechanisms (robots, mechatronic systems) based on a new type of movement is developed by scientists and engineers. Let us consider some examples of such systems in terms of design.
One of the first papers to study dynamic systems with moving internal masses is the paper of Chernous’ko (2005). The considered mechanical system consists of a solid body that moves horizontally along a straight line and an inner body that moves relative to the solid body along an axis parallel to the axis of motion of the body. The same design is used in the paper of Chernous’ko (2006) for more detailed analysis. And the continuation of the investigation of this mechanism is given in the article of Chernous’ko (2008), but we will not go into details, leaving it to the reader’s discretion. Nevertheless, this mechanical design also attracts Fang et al. (2011) and Bolotnik et al. (2012). So we can confidently say that the system of this design is well studied.
In the work of Bolotnik (2006) the design of a mechanical system differs from the design in the paper of Chernous’ko (2005) in that the internal mass moves not only along the horizontal line, but also along the vertical, so the inner mass moves in a vertical plane.
In the work of Chernous’ko et al. (2013) the design of the mechanical system is as follows: an articulated mechanical system consists of a rigid body (the main body) and two identical rigid links, attached to the main body by revolute joints. The system moves in a resistive medium in a horizontal plane.
In the paper of Bizyaev et al (2014) a spherical shell with a moving rigid body inside is investigated. Internal body is fixed inside the shell by means of two different mechanisms.Paper of Ivanov (2014) deals with the motion of a cubic rigid body (so called M-block) with internal rotor. The M-block motion is caused by a sudden brake of the rotor, leading to a jump or rolling of the body.
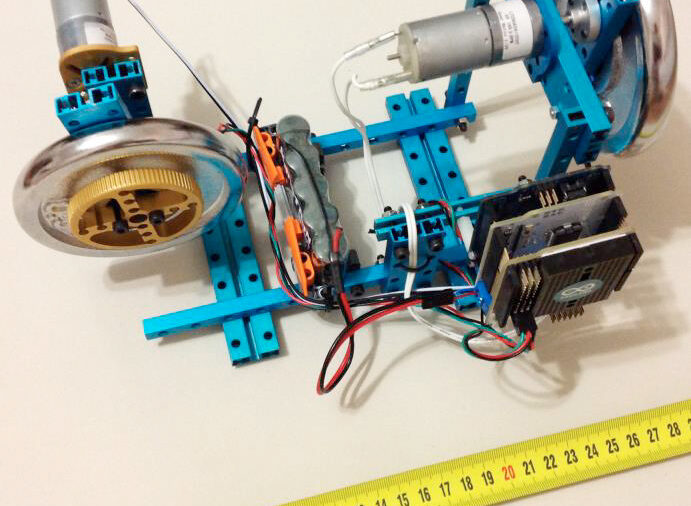
Sakharov (2015) considers a rigid body, which is a hollow parallelepiped. Two material points move inside the body along the sides of the mechanism. In our previous work (Semendyaev et al. (2016)) we consider solid system consisting of a rigid body with two internal masses (in the form of eccentrics– unbalanced massive disks). Eccentrics rotate with variable angular velocity and have no contact with external medium. The body has a contact with a horizontal rough surface with dry friction and moves short steps (or slides) in plane due to rotatingeccentrics.Previously (Semendyaev et al. (2016)), the translational case of motion of the mechanical system is investigated. In this paper the rotational case of motion of the rigid body (in the form of tripod) caused by rotation of the transverse eccentricis considered.

Читать статью Solid System with Two Massive Eccentrics on a Rough Plane